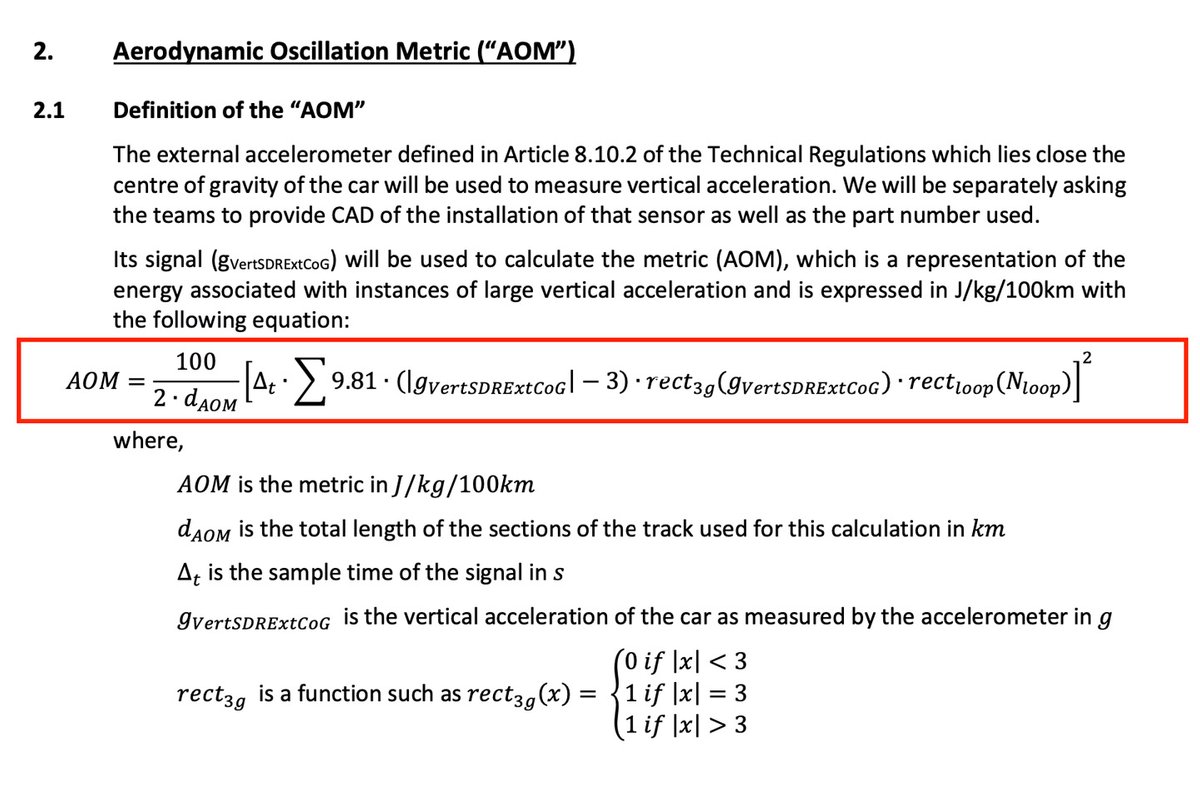
The metric seems to be based on kinetic energy and work but I'm a little too thick to understand the exact derivation right this moment, lol.

It doesn't say what the summation is over (presumably N_loop), what
x is, or what rect_loop is? It doesn't matter what the time spacing between those N_loop number of values is, just that they are covered by a time period of Δ_t?
Edit -- Oh, ok. In the brackets there is time * (acceleration IF acceleration > 3g), which gives units of s*m/s^2, which gives m/s. Then this is all squared, which gives m^2/s^s or the units of v^2.
Kinetic energy is 1/2 * m * v^2.
So 1/2 appears in the AOM metric, but mass is missing so kg is divided out of the units of AOM, giving units of J/kg to start with. Then the multiplication factor 100 / (distance in km) is applied which gives the equivalence per 100km, i.e., if this happened over d=10km, then multiplying by 100/d means it would happen 10 times that over 100km, giving AOM its final units of J/kg/100km.
Ok, that makes sense.

n_anirudh wrote: ↑02 Jul 2022, 14:14
What is N_{loop} or rect_{loop}?
I dont understand where or how the "-3" comes in, Also can someone explain as to why this is averaged over distance. Isnt peak loads what we are looking for?
It seems redundant, they both subtract 3 but then give all the values less than 3 a multiplication coefficient of zero as well. I guess they are using 3g as the mean acceptable acceleration, and they don't want it to cloud the final metric hence subtracting that level of acceleration away from contributing to the metric?
Edit -- I think that |
x | is meant to be |
g_(vert etc.) | as otherwise it doesn't make sense.
Edax wrote: ↑02 Jul 2022, 11:01
I am surprised by the simplicity of this equation.
If I look at the footage there is bound to be some frequencies that are much more damaging than others.
Yes, the metric is very much a Fourier transform free zone, lol!

It seems they are going for a simple time- and distance-averaged kinetic energy average of only when g exceeds 3g and that's all.

TLDR: The FIA directive for porpoising/bouncing puts a limit on accelerations over 3G.