Suspension kinematics...

[riff_raff]

n smikle,

What is probably more important than the static camber value at set-up, is the camber gain during suspension travel. The relative positions and lengths of the upper and lower a-arms and pivots mostly determines the camber gain/loss rates during suspension travel.

Of course, the tire sidewalls absorb more suspension travel than the actual suspension itself. I don't know the actual spring rates of a modern F1 tire carcass, but several years ago the spring rate I heard quoted for a radial F1 race tire was about 2500 lbs/inch.

Hope that helps.
Terry

[marcush.]

The Man,

If you're bonding to carbon composite, the metal of choice (due to galvanic compatibility) is normally titanium. Plus, titanium alloy has properties that make it an excellent choice for the bending stress/fatigue loading conditions that an A-arm flexure requires.

The reason flexures are preferred for A-arm attachments, is that they eliminate the varying joint friction that spherical bearings inherently have. This makes achieving a consistent suspension set-up easier, since the friction and dampening effects of the spherical joints is eliminated.

Regards,
Terry

one has to understand using two bearings to attach a A-Arm to the chassis creates a lot of friction as soon as all bolts are tightened,especially if you put the sphericals horizontally you will effectively not have a possibility to have a fixed and a floating bearing arrangement -effectively this will result in a situation you will always face some bind until the bearings wears (and as a result of wears develops slackvery quickly),so this is on the engineering side not a good solution.
with the flexures ,I heard of some issues with buckling forces/instability under braking (MGlola LMP675) which led to development of lower A-arms with conventional spherical bearings so you have to be careful not to make those flexures too long /too thina nd of course watch for the fatigue ...

[PlatinumZealot]

n smikle,

What is probably more important than the static camber value at set-up, is the camber gain during suspension travel. The relative positions and lengths of the upper and lower a-arms and pivots mostly determines the camber gain/loss rates during suspension travel.

Of course, the tire sidewalls absorb more suspension travel than the actual suspension itself. I don't know the actual spring rates of a modern F1 tire carcass, but several years ago the spring rate I heard quoted for a radial F1 race tire was about 2500 lbs/inch.

Hope that helps.
Terry

In my calculations, I have the camber gain relative to the chassis; I can dial in a rotation for each lower A arm and the camber relative to the chassis comes out. I am Ok with this part. This is just for a floating chassis though. Then now I put on tyres and lower the car onto the road mathematically. This is so I can get the camber of the wheels relative to the road itself.

To do this i wanted to get the height from the centre of the wheel to the road.
Since tyres are not shaped like a sphere, I have it that the height from the road to the centre of the wheel changes when the tyre is rocked left or right depending on the shape of the tyre cross section. (like rocking an egg). Still, I have second thoughts about this because of the compressibility of the tyres.
Since the squashing of the tyres stops the tyre from going on its edge I don't know how the final tyre height is affected.

Example here to see what I am trying to say:




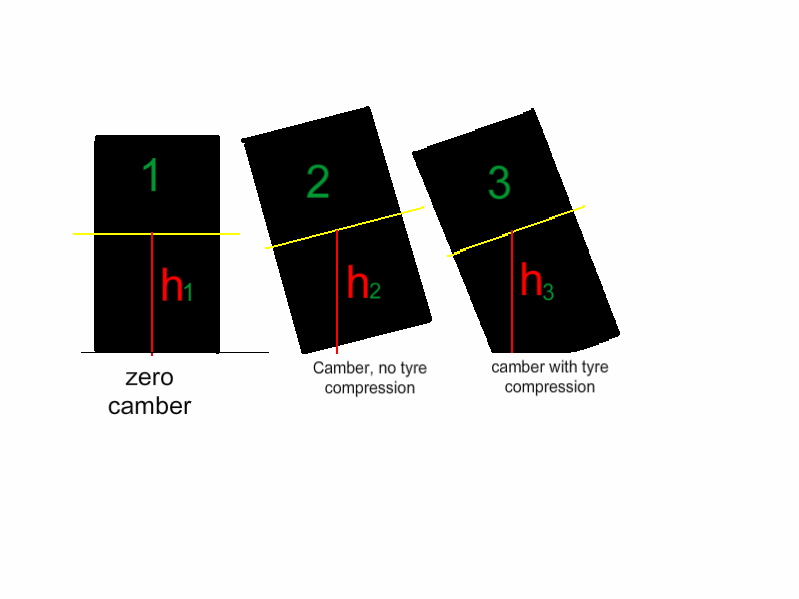
How would I use the tyre spring rate(s) to find h3?

I have a feeling it has something to do with the lateral stiffness of the tyres (which I have no clue about)
Or some centre of bouyancy/pressure thingy.. i have no clue about those areas either if that is the case. Is it even necessary?

Reminds me of squashing a balloon

[Jersey Tom]

You can't determine that without good tire data. Tire vertical spring rate changes with just about everything.. load.. camber.. inflation..

[autogyro]

You can't determine that without good tire data. Tire vertical spring rate changes with just about everything.. load.. camber.. inflation..

It might be interesting to think about what happens when the rain comes to effect the computer results you have.
Or for that matter when a car hits a high curb (kerb).

[Belatti]

There's nothin to read, man. It's a beam that bends. Simple concept. I have a flexure A-arm off a Lola champ car.. the flexures add surprisingly little to the wheel rate.

Can post or send me a close pic of that?

[PlatinumZealot]

You can't determine that without good tire data. Tire vertical spring rate changes with just about everything.. load.. camber.. inflation..

Looks like I have to buy a book about Tyres then...
What do you suggest as a temporary solution? (This height is what is stopping me from completing my kinematic model of my car). Assume a constant tyre radius? What is the order of inaccuracy to expect? A few milimeters?

[Jersey Tom]

Can post or send me a close pic of that?

Next time I dig it out. It's really pretty simple though.. just a strip of metal with a hole for a bolt, in place of a spherical or rod-end bearing.

You can't determine that without good tire data. Tire vertical spring rate changes with just about everything.. load.. camber.. inflation..

Looks like I have to buy a book about Tyres then...
What do you suggest as a temporary solution? (This height is what is stopping me from completing my kinematic model of my car). Assume a constant tyre radius? What is the order of inaccuracy to expect? A few milimeters?

Inaccuracy.. depends on a lot. Could be hardly anything, could be huge.

Really depends what you're going for in your vehicle model. Could assume the tire is rigid (constant radius) .. could assume a constant tire rate..

[PlatinumZealot]

Ok let me bring this back on topic.

What is the goal of the kinematic design of the suspension?

[Jersey Tom]

Ok let me bring this back on topic.

What is the goal of the kinematic design of the suspension?

To (dynamically) orient the tires in such a manner that provides the vehicle handling parameters / metrics that you want.

[tok-tokkie]

Just a sideline to the flexures.
Pendulum clocks use flexures for the suspension & articulation of the pendulum. On a conventional 1 second pendulum grandfather clock that is 17 280 flexings each day of about 2.5 degrees each side of vertical. They last for years, are very small and the pendulum bob is not insignificant.

[marcush.]

not what YOU want ,but what the tyre wants !
If you base your layout on false assumptions or wrong basics the kinematics sure will do what they are designed to do ,but will this result in better carperformance ?

To (dynamically) orient the tires in such a manner that provides the vehicle handling parameters / metrics that you want.

so to stick on a different type of tyre should never ever result in better performance as the kinematics should bee not optised for this tyre.
If sticking on a different tyre does indeed result in better performance ,your old
tyre was either complete crap or you ran with wrong kinematics ,setup,weight distribution,etc.

And you don´t forget not only kinematics (theoretical) is the key but real dynamic numbers ,which take into account :flex in rim,hub etc -installation stiffness- chassis flex - free movement of parts (stick/slip/bind/loose fit) could all stirr up everything and convert all the good ideas to a complete mess.

[Mystery Steve]

...to stick on a different type of tyre should never ever result in better performance as the kinematics should bee not optised for this tyre.

What exactly would you be optimizing? Optimization implies a maximizing or minimizing a variable at the expense of the other variables. A well designed suspension should work well regardless of the tire.

Jersey Tom: I've never seen tire data personally. Is there any way you can qualitatively describe what generally changes between different tires? Obviously, different tire contructions would produce different results, but do the different compounds, of the same construction, drastically shift the peaks on the various plots or is it usually just scaled, or both?

[RacingManiac]

You should design always from the tire up. Which means the geometry should feed the tire with its desired characteristic for best grip. Some tires will like more camber, others less, some will be pro ackermann, others anti. So you design your kinematic to match that. Which means if you did it properly, swapping one to another will mean that the new tire may not be performing at its best. Now there is every possibility that it might do as well as it did before, but relative to itself, it may not be performing to its best....

With our level of monkeying around for FSAE cars I don't think we've used any tires that has been that demending yet. The most obvious difference has been pressure difference, which is easy enough to change, and we've built in enough adjustment for camber or even ackermann to adjust setup. But we've never that closely to analytically design suspension to the tire curves. But I'd imagine at the top level(or other better school's level) motorsport that should be the approach to doing things....

[RacingManiac]

You can't determine that without good tire data. Tire vertical spring rate changes with just about everything.. load.. camber.. inflation..

and age and heat cycle too I think....