Technical F1 Explained wrote:
Slip Angle
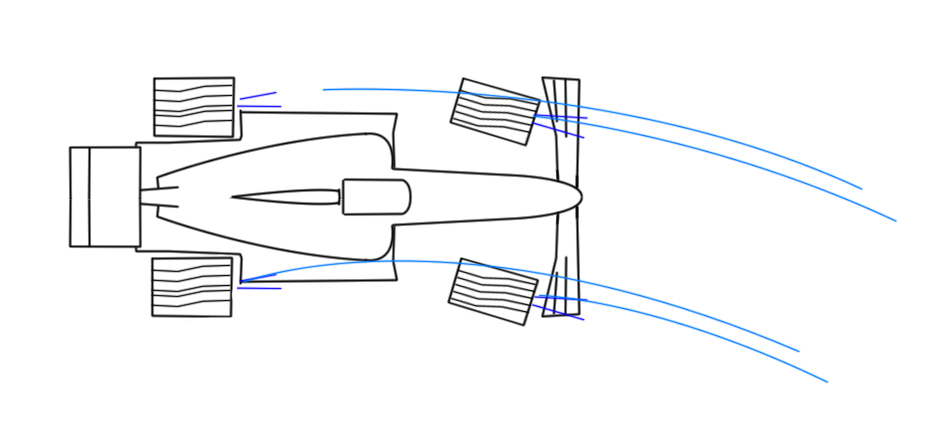
The slip angle of a pneumatic tire can be defined as the angular displacement between the plane of rotation of the wheel (the direction in which the rim is pointing) and the path that the rolling tire will follow on the track surface. For the vehicle to change direction, regardless of road speed, each of the 4 tires of the vehicle must have some slip angle.
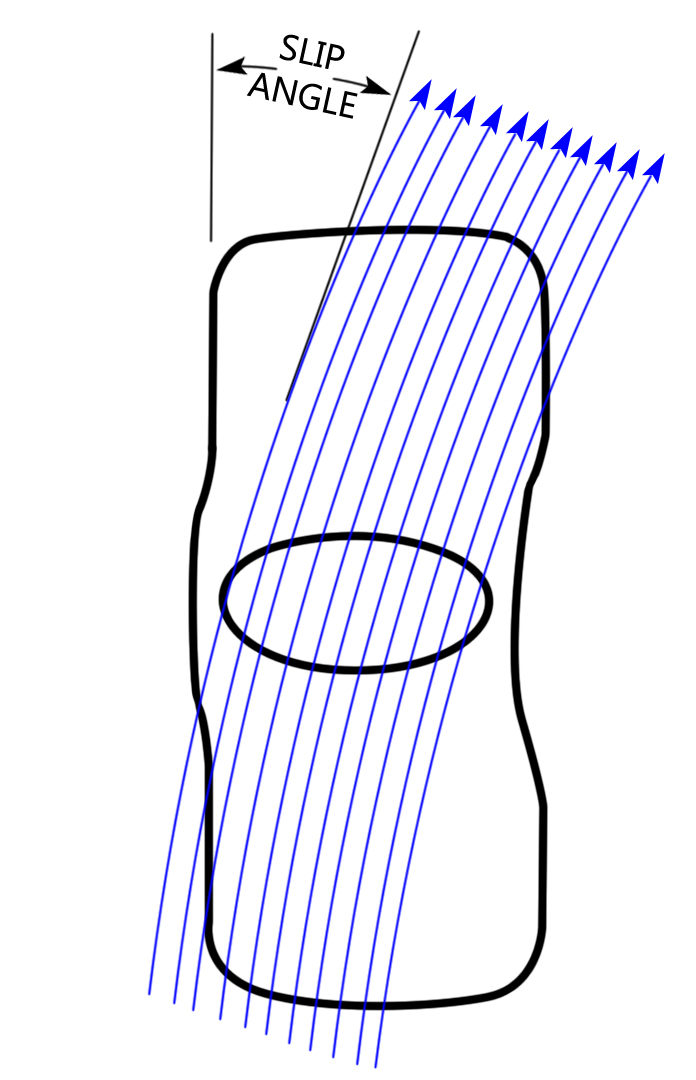
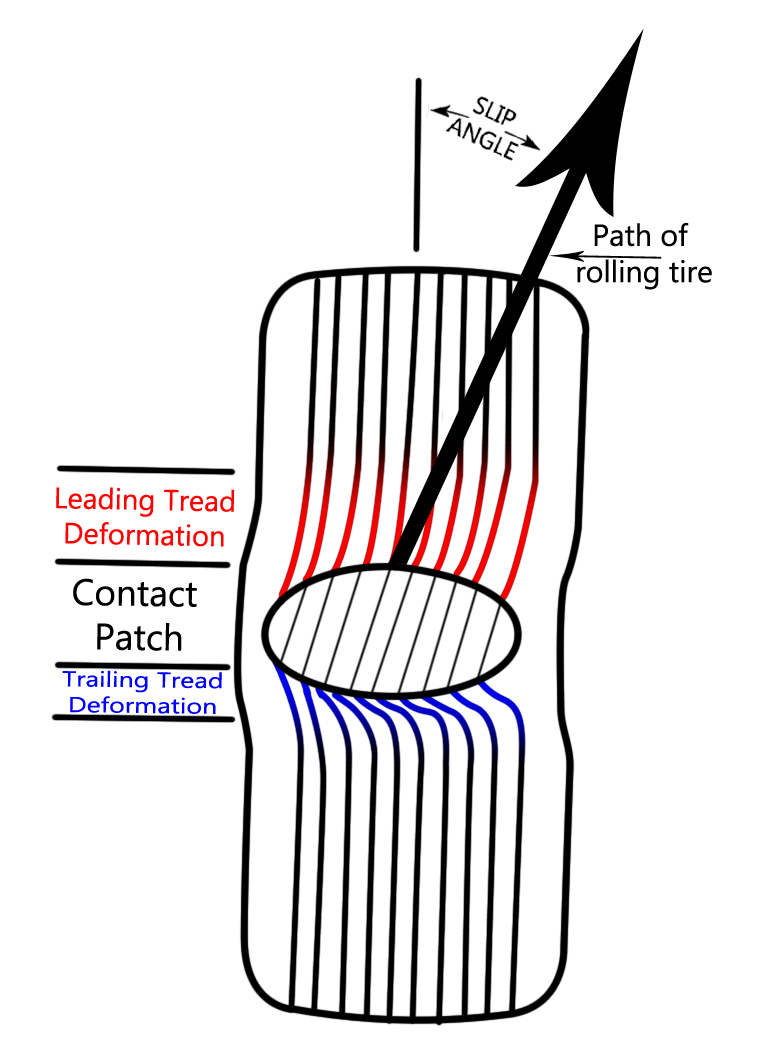
The pneumatic tire is elastic in twist and because of this property exists the slip angle phenomenon. When the tire is turning, the segment of the tire that is in contact with the track surface will resist the turning moment and will not turn as much as the rim does since it is elastic. The rolling path on the road surface will always lag behind the plane of rotation of the rim by some value of angular displacement which is the value of slip angle.
Visualize a single particle of rubber on the tread. Most of the time, it is not in contact with the track surface. However, when the particle in question does roll into contact with the track, progressing form the leading edge of the contact patch to the contact patch itself and then to the trailing edge of the contact patch, that is when the elastic deformation takes place. Although the actual deformation takes place during the time that the particle is actually in contact with the track, all of the particles are interconnected so displacement begins before the particle is in contact with the track surface. Due to the properties of rubber, the leading tread deformation is gradual while the trailing edge deformation is much more sudden.
In order to proceed with the relationship between slip angle, coefficient of friction and the cornering force, it has to be noted that grip from a racing tire is developed by a combination of mechanical gripping on the road surface irregularities and by molecular adhesion between the tread surface and the track surface. The molecular adhesion itself comes into play at very high loads and coefficients and it is the reason behind being able to leave black marks on the track when the car is neither spinning or locking its wheels or sliding.
Slip Angle, Coefficient of Friction and Cornering Force
Coefficient of friction varies with slip angle; subsequently the cornering force will vary with slip angle as well. The coefficient of friction will increase as slip angle increases until a given point where any further increase in slip angle will result in a decrease of Coefficient of friction resulting to the tire “breaking lose” or losing traction.
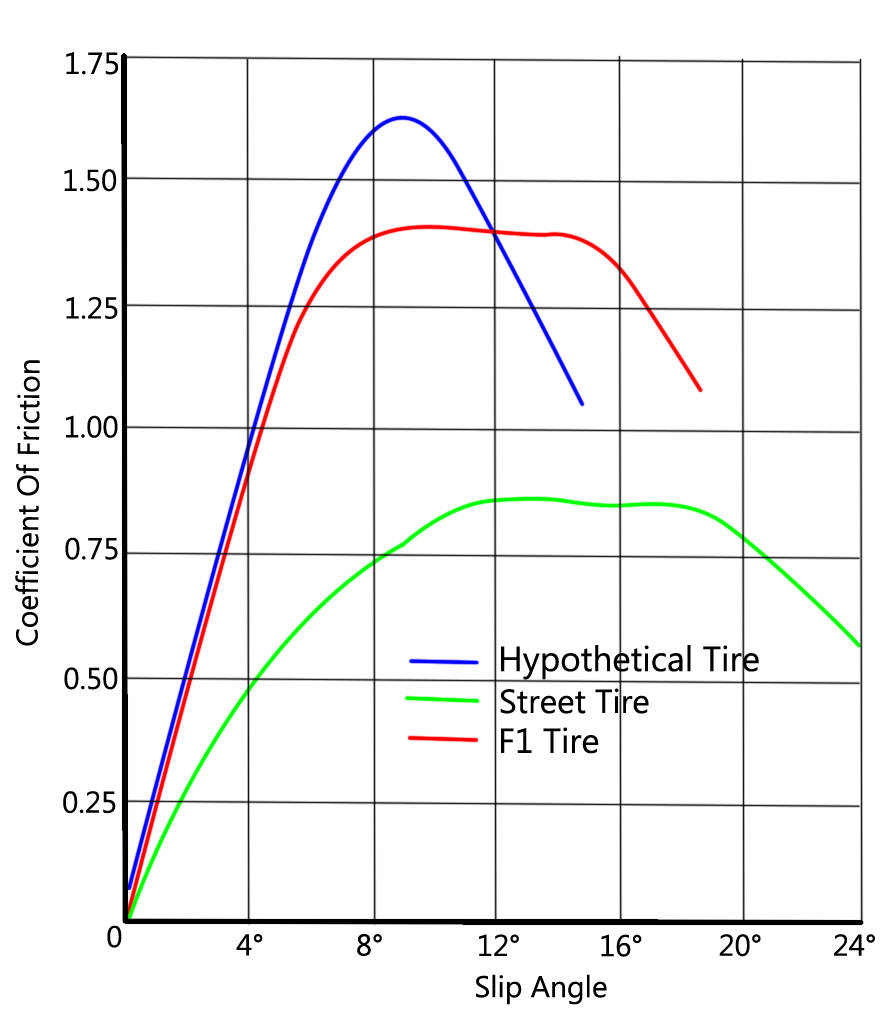
An ideal tire will give a linear, high gradient curve at the beginning so that the coefficient increases rapidly (so that the driver can build up cornering force quickly) with increasing slip angle and then levels off gradually with a reasonably flat curve for the widest range of slip angles possible (for the driver to be able to get as close as possible to the limits of adhesion). The segment of the curve at the top where an increasing slip angle does not change considerably the coefficient of friction is called the threshold range. The expected downward slope of the curve has to start off gently so that the driver can feel it and react accordingly. If the curve would plunge down suddenly then the driver would spin off the track. For example if the curve of the tire would look like the hypothetical tire curve, even the most daring, experienced and talented drivers would not be able to exploit the maximum potential of the tires. A tire with this graph would be a very, very inefficient one. In reality what happens at the point the curve starts sloping down, the tire is running out of elastic capability and is starting to slide, a further increase in slip angle will get the car into a bigger slide while the elasticity of the tire would continue to decrease. A high slip angle will generate more heat than a low slip angle. If heat is generated beyond what is needed to get the tire into its optimum operating temperature, the tire deteriorates and there is no contribution to performance.
Vertical Load VS Coefficient of Friction
A tire’s coefficient of friction decreases as load increases. However, this does not mean that traction decreases. Traction will increase with an increasing vertical load.
This effect is illustrated in the graph below:
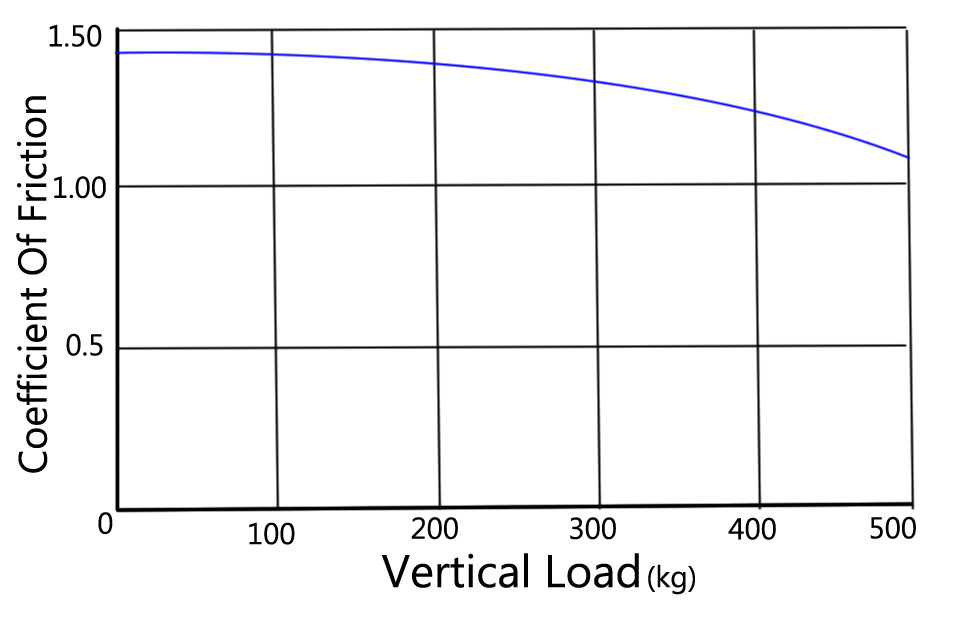
To explain this in simple arithmetic, let’s assume each rear wheel had to support a load of 200 kg, using the formula described in Part1 (ForceOfFriction=μ CoefficientOfFriction x Normal Reaction), 1.35 x 200 kg = 270 N, times 2 for both rear wheels gives us 540 N. However if we add 50 kg per wheel because of load transfer, then (1.33 x 250) x 2 = 665 N and if we add the effect of the rear wing, which for example gives 200 kg of vertical load to each rear wheel, then (1.26*450) x 2 = 1134 N. So despite the decreasing Coefficient of Friction, the force developed increases as vertical load increases. The truth is it is not that simple, but the point is that increasing vertical load decreases the Coefficient of Friction and increases the traction capability of the tire.









