Hello all.
The “JetMan-Dubai” team claims 400Km/h top speed for “their” Rossy’s DeltaWing.
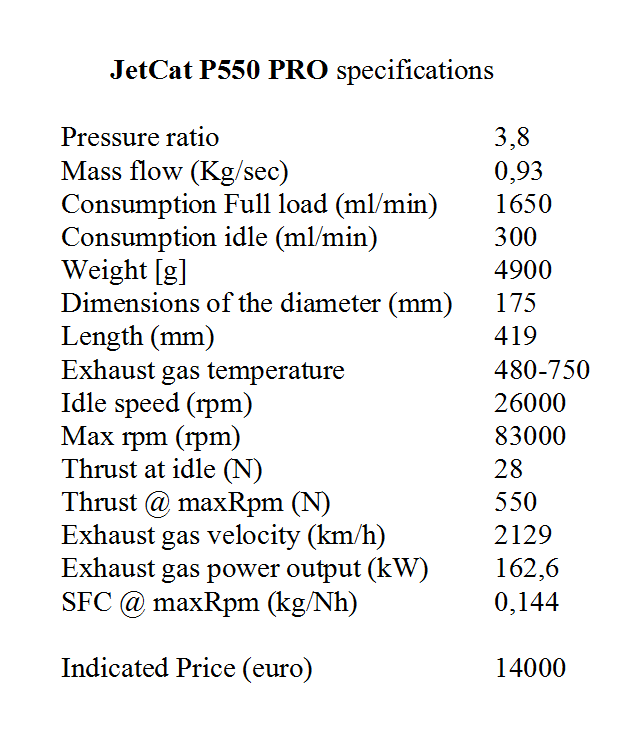
Based on the JetCat P550 specifications, let’s see what the various numbers have to “say” and how they connect with each other:
With 400Km/h (111m/sec) speed and 2,200N total thrust, the power that pushes the DeltaWing forwards is:
P = 111m/sec * 2,200N = 245kW
The energy E provided per hour by this power is: E = 245kWh.
Supposing a specific gravity of 0.8Kg/lit for the fuel,
the 4*1650ml/min = 396lit/h fuel consumption per hour of the four jet turbines at full load gives a “BSFC” (Brake Specific Fuel Consumption), if we can call it so, of:
396lit * 0.8Kg/lit / E = 316Kg / 245kWh = 1280gr/kWh
and the resulting “BTE” (Brake Thermal Efficiency), if we can call it so, is:
80g/kWh / 1280gr/kWh = 6%
Where the 80g/kWh comes from?
80gr/kWh is the BSFC of an ideal engine running at 100% BTE;
When a giant marine low-speed 2-stroke Diesel engine consumes 160gr of fuel per kWh of mechanical energy produced, it operates at 80/160 = 0.5 = 50% BTE.
The total Exhaust Gas Power Output is 4 * 162.6kW = 650kW, while the power that actually pushes the pilot / DeltaWing is, as previously calculated, P = 245kW.
That is, the four jet turbines provide 650kW + 245kW = 895kW of power from which the 27% ( 245 / 895 = 0.27, i.e. about one quarter) pushes the pilot / aircraft forwards, while the rest 73% (nearly the three quarters) is lost in the exhaust gas.
This means that the jet turbines run at 22.5% BTE ( 80gr/kWh / (316Kg/h / 895kWh)= 0.225).
22.5% = 6% + 16.5%
The 6% of the fuel energy pushes the aircraft forwards,
the 16.5% of the fuel energy is lost with the high speed exhaust gas,
while the rest 77.5% of the fuel energy is never transformed into “mechanical” (or “aerodynamic” etc) energy.
In the JetCat P550 specifications the Exhaust Gas Power Output is given as 162.6kW;
Taking the derivatives of both sides of the kinetic energy equation:
E = (1/2)*m*v^2
it results:
dE/dt = (1/2) * dm/dt * v^2.
(the dm/dt is the mass flow; the speed v of the exhaust gas is constant 2129Km/h).
(1/2) * (Mass Flow) * (Exhaust Gas Velocity)^2 = 0.5 *0.93Kg/sec * (2129Km/h)^2 = 0.5 * 0.93Kg/sec * (591.4m/sec)^2 = 162.6kW, which is the Exhaust Gas Power Output given in the specifications.
In the P550 specifications the Specific Fuel Consumption (Kg/Nh) is given as 0.144;
0.144Kg/Nh = 1650ml/min * 60min/h * 0.008Kg/ml / 550N
(0.008Kg/ml is the fuel specific density, 1650ml/min is the fuel consumption at full load).
For the pilot, who is “in the open air”, the 400Km/h speed seems exhausting, if not unaffordable for more than a few seconds.
At lower speeds, say at 200Km/h, a substantially smaller portion of the power provided by the turbines is utilized to propel the aircraft, while the rest power provided by the turbines is lost with the exhaust gas; however the aerodynamic resistance falls more (it falls with speed square).
The lower the speed, the smaller the portion of the power provided by the turbines that is actually used to propel the aircraft, with the rest power being lost in the exhaust gas (in case of zero speed, while the turbines continue to consume fuel, all the energy they provide goes to the exhaust gas).
The higher the speed, the more of the turbine power goes to propel the aircraft, and the less power is lost in the exhaust gas.
Quiz:
Suppose Rossy wants to cross Calais channel (some 22miles / 35Km) with his new DeltaWing.
At which speed the required fuel is minimized?
According the previous,
at normal / affordable speeds, say from 100 to 150mph (160 to 240Km/h), only 3%, or so, of the fuel energy is propelling the Jet-Pack Flying Device; the rest fuel is burnt to accelerate the exhaust gas and to warm the atmosphere.
Even if the CO2 footprint was not an issue, the running cost gets extreme.
Worse even, the weight of the required fuel for a useful (say, 50Km) range becomes too high, and this weight has to be lifted by the JetPack Flying Device (just like heavy batteries have to be lifted by the electric Flying Devices).
In comparison, the Portable Flyer with its internal combustion engines and its propellers is many times more efficient than the JetPacks, it needs many times less fuel for the same range, it has many times longer range with the same quantity of fuel, it has many times smaller CO2 footpinrt, it has many times lower running and ownership cost, etc.
Thanks
Manolis Pattakos