Well, I have 15 minutes after lunch, a coffee and some peace of mind. Let's use it for the forum.
Marekk, you claim that in a bicycle wheel all the spokes work. Now I see the problem we have. I assumed you had certain knowledge, my fault. So, let's take the long road here. I'll write quickly (thanks heaven I can type).
This should be a long explanation. Here you have a regular concrete-steel beam:
Concrete beam under load. It twists at the center.
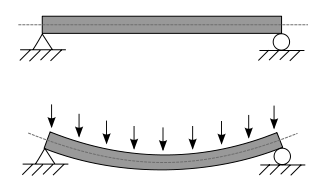
As you can see, under load, the beam is stressed in the lower part (it stretches), while it is compressed in the upper part (it shortens). Thus, when you make a concrete beam the steel is located in the lower part of the beam. Concrete is poor under tension, while steel is good. The converse is true: steel rods are useless under compression while concrete is good.
Stresses at the center of a beam: the top is in compression, bottom in tension. Hence the momentum at the center that makes it sag. At the supports you have only shear (not shown) caused by the vertical loads in the abutments. This torque is maximum at the center of a simply supported beam.
This, on the other hand, is a pre-stressed beam. It seems the same thing, but it's not (in the same way a balloon tyre is not the same as a regular beaded tyre). Here, the steel cables have been tensioned. You can see how the beam is curved upwards because of that stressing (the cables are taut). Now the load is carried by the concrete, because ALL OF IT is under compression. The cables pre-stress it, so when you put a load on top of it, parts of the concrete relax (they lose part of the compression) and other parts increase the compression. This creates the same momentum (a par or torque) that is what resists the load forces. If I drew the same stress diagram, it wouldn't look like the two triangles I showed before, but it would look like a parallelogram. The bottom would have more compression under load than without it, while the top, even under compression, would have less.
Pre-stressed beam
 Stresses in a prestressed beam
Stresses in a prestressed beam

Notice you use the magnitude of those stresses to DESIGN THE DIMENSIONS OF THE BEAM. They are not supporting the bridge, those forces are vertical and at the abutments. You make this analysis to know how thick is the beam and the cables inside, but they are not what you analyze when you make an static analysis of the beam.
On a bicycle wheel, the same thing happens. You can make a regular spoked wheel, for example of wood. When you load it, the lower spokes resist the load through compression. End of the question.
Now, a bicycle rim has a profile with very low resistance to torque. If you take a rim without spokes and try to bend it (with a torque or momentum), you can do it with your bare hands.
So, enter prestressing: you use spokes that are stressed, with hundreds of kilos, to do the same thing as in a prestressed beam, only that the beam is not straight, but in a circle. This rim, that behaves so poorly under tension or torque, now is working under compression in the tangent direction (tangent to the rim) and under radial shear (compression you know what it is, while shear is the same force you make when you break a chocolate bar: perpendicular to the surface of the rim). For this compression and shear, aluminium is very good. It is very hard to compress the rim in a tangential direction and you can try to shear aluminium with your hands: you cannot. However, the bending moment has been "replaced" by those forces, in the same way that a pre-stressed beam has all concrete under compression to avoid tensioning it (it would break if tensioned).
Thus, the profile that few minutes ago, without spokes, was bendable, now can take the load of a huge person. The calculation of those internal forces allow you (as in a prestressed beam) to calculate the profile you need in the rim and how thick the spokes should be. As in the beam, THOSE FORCES ARE INTERNAL. They are not supporting the bike, but the rim.
When you sit on yor bike, it happens, essentially, the same thing that happens in a prestressed beam: ALL THE SPOKES ARE UNDER TENSION (Edit: I wrote COMPRESSION initially, my mistake. Thanks Tim for pointing this out), with hundreds of kilos each. If you "sum" (not like vectors, but in magnitude) all the forces in the spokes you can end easily with a force of tons. THIS FORCE IS NOT SUPPORTING YOU, IS MAKING THE RIM RIGID.
I hope everybody has followed me until this moment.
Now, when you sit on your bike, the lower spokes lose some tension, while the upper spokes gain some tension. I say "some" because your weight (a few tens of kilos) is relatively small compared with the forces that are making the rim rigid.
This is, in a way SIMILAR to what Tim Wright said. However, it's not the same as what I understood he said. The forces in the spokes are making the beam (well, the rim) rigid. The car (or your butt) is actually supported by this small increase in tension in the upper spokes. This causes a (slight) tension lose in the bottom spokes. The rest of the forces, beside this small increase in tension (60 to 80 kilos, compared with 1.000 or more kilos used in the prestressing of the spokes) from an static calculation point of view, DO NOT EXIST. They are there only to make the rim rigid.
If you say they are playing a part in the static equilibrium of the spoked wheel, it would be as claiming (and calculating) the molecular forces between the atoms of wood that make a wood tyre rigid. When you speak of a wood spoked wheel YOU DO NOT TAKE IN ACCOUNT THE FORCES BETWEEN THE ATOMS IN THE WOOD. THOSE ARE INTERNAL TO THE MATERIAL AND IT WOULD BE WRONG TO DRAW THEM OR TAKE THEM IN ACCOUNT IN A DIAGRAM OF FORCES IN THE WHEEL. Certainly, in a spoked bicycle wheel, those tension (again, I wrote compression initially, my mistake) forces, that, I repeat, are there to make the rim rigid, DO NOT CARRY YOUR BUTT.
A Formula One tyre works the same: the air is making the tread rigid. The ACTUAL WEIGHT OF THE CAR is supported by the walls at the top. The rest of the forces, which are radial and CANCEL MUTUALLY (one side of the wheel is being pushed outside by the air, but is compensated by the diametrically opposed side) ARE THERE ONLY TO MAKE THE TREAD RIGID. Hence the picture I made. I hope this is clear.
OF COURSE you can claim that those forces transfer the load, but, I assure you, this phrase is totally nuts (not you, but the phrase).
It would be like, I don't know,
assuming that the Planet Earth is what transfers the cars around the track, so, if you want to know the speed of the cars, FIRST you have to calculate how fast the Earth move in space, and FINALLY arrive to the correct speeds of the cars by calculating the difference between their speeds around the center of the Galaxy and the speed of the Earth.
If I write a post calculating top speeds at Spa, and I start by calculating the speed of Earth around the Sun, people would look at me and say: "Ciro, what did you smoke this morning?".
In the same way, the walls at the bottom do not carry the weight of the car. Is nuts to create an static diagram that shows all forces on the walls, and FINALLY subtract the force in the lower walls from the force in the upper walls to arrive to the correct force diagram (which is the one I drew). Those INTERNAL forces you use when you calculate how thick is the tyre, not how the forces between the axles and the tyres are. Got it?
You also ask how a car moves forward. We had a very detailed thread on it. Now, I have to work, this evening I will try to look for it ans summarize it here.
Finally, I say again: torsional stiffness is treated in Pacejka book, Chapter 9.4.2, I don't have it at hand but I have good memory. They are from 30 to 100 hz. Please, read the book (or somebody who has it at hand, please, give us some ballpark figures).
End of discussion as far as I can go. If you get it, you get it. If you don't get it, you don't get it. I do not like to repeat arguments. "Treads" become boring then (ha, ha! What? Did I already said that? Impossible... am I repeating myself? Funny, isn't it? No? Well...).
BTW, even the Wikipedia article on spoked wheels suffers from the same confusion it seems to me you have. Half the references are written by guys that clearly do not have the slightest idea of how a structure works, so the article ends affirming (sigh) that half the people thinks that the lower spokes in a bicycle wheel actually support the weight. If you think so, well, good luck with that, and please, explain to me the buildings they have designed, so I won't enter them, specially during an earthquake.






